The Risks of U.S. Sovereign Debt
From February 1st through the 4th, the Buttonwood's notebook blog of the Economist magazine had some interesting posts on the risks of sovereign debt. They were titled The debt trap: ranking the suspects, More debt rankings, The new gold standard, and Adding in the deficit. The first post addresses the effect of a debt trap, where the bond yield is higher than the economy's nominal growth rate. The second post adds in the effect of the debt-to-GDP ratio. Finally, the last post adds in the effect of the primary surplus/deficit. Also called the primary balance, this is equal to revenues minus spending, excluding interest costs. If the primary balance is positive, it's a primary surplus. If negative, it's a primary deficit.
The effect of these four factors (bond yield, GDP growth, debt-to-GDP ratio, and primary balance) are also addressed in a Reuter's column titled Sovereign debt maths show risk of vicious circle. The column mentions all four factors in the following excerpt:
To see how these play out, consider two countries. One has a moderate debt load, 50 percent of GDP, which carries a 4 percent average interest rate. If the budget is in primary balance, the government will still run a deficit of 2 percent of GDP, which is 4 percent (the interest rate) of 50 percent (the debt). As long as nominal GDP grows by 4 percent, the ratio of debt to GDP stays the same.
The validity of this final statement (that the ratio to GDP stays the same) can be seen via the following formula:
The value of 1.04 in the above formula is of course the correct factor to calculate a 4 percent increase and is equal to (4/100) + 1. In any case, the column goes on to describe how this same formula applies to the country with the high debt load of 100 percent of GDP. It then continues:
But the equilibrium is fragile. It can be disturbed in three ways: nominal GDP growth can decline, interest rates can go up or the country can start running a primary deficit. The pain is much worse for highly indebted countries like Greece, which has managed all three at once.
Start with growth. Imagine nominal GDP growth drops to zero. If nothing is done, the debt/GDP ratio will rise by 2 percentage points in the moderately indebted country, but by 4 percentage points in the highly indebted one.
This situation can be represented by the following formula:
For the moderately indebted country, this will equal 1.04 * 50% of GDP which equals 52% of GDP, an increase of 2% of GDP. For the highly indebted country, this will equal 1.04 * 100% of GDP which equals 104% of GDP, an increase of 4 percent of GDP.
The column continues:
Countries can keep that key ratio from increasing, by running compensating primary surpluses. That means moving from balance to a surplus of 2 percent of GDP for the moderately indebted and from zero to 4 percent for the heavily indebted. The higher the debt level, the more the government’s belt will have to be tightened.
To verify this, one can first calculate the formula for the primary surplus in the case where the debt to GDP ratio does not increase as follows:
For the moderately indebted country, the necessary primary surplus will be
and for the highly indebted country, the necessary primary surplus will be
Hence, the above formulas show that countries with different levels of debt to GDP can maintain those levels as long as GDP growth keeps pace with the average interest rate and the countries run primary balances of zero. However, they also show that, if one or more of these factors fall out of balance, the country with the higher debt load will see greater negative effects.
The following graph shows the net interest on U.S. Treasury securities as a percentage of outlays, debt, and GDP, along with the nominal growth in GDP:
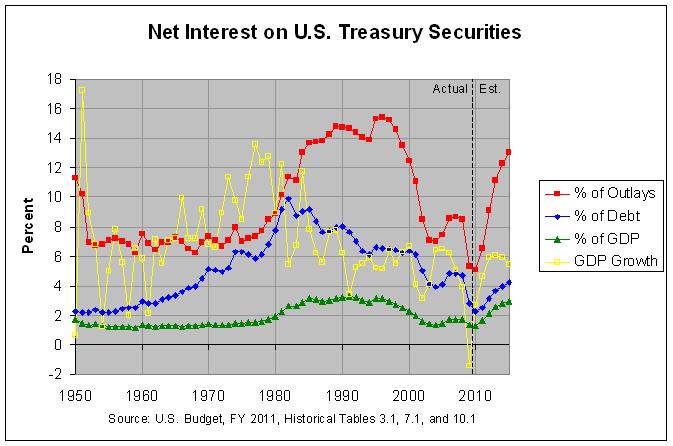
The actual numbers and sources for this graph can be found at this link. The net interest as a percentage of debt (the blue line) should give the average interest rate being paid on U.S. debt held by the public. As can be seen, the GDP growth rate was generally above the average interest rate from about 1951 to 1981 but was generally below the average interest rate from about 1982 to 2002. From 1951 to 1981, the average interest rate was about 4.2%, nearly half of the average GDP growth rate of about 8.2%. From 1982 to 2002, however, the average interest rate was about 7.3%, over a full percentage point more than the average GDP growth rate of about 6.1%. Hence, it is possible for the average interest rate to surpass the average GDP growth rate for an extended period of time.
The following graph shows the primary and unified surplus or deficit(-) of the U.S. budget:
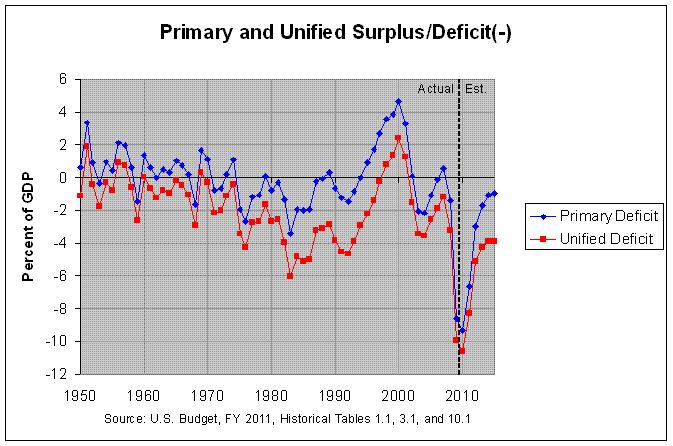
The actual numbers and sources for this graph can be found at this link. As can be seen, the primary surplus appears to have been, on average, close to balance since 1950, at least until recently. In fact, from 1950 through 2007, the average primary surplus was about 0.14 percent of GDP. However, the graph shows that the primary surplus became a huge deficit in 2009 and is projected to just recover to about a one percent of GDP deficit by 2015. In addition, the tables at this link show that the primary deficit is projected to rise sharply over the coming decades. These numbers come from the most recent U.S. Budget and project that the primary deficit will reach a massive 24.3 percent of GDP by 2085.
Hence, the large primary deficits projected over the long-run would seem the most obvious risk to U.S. sovereign (government) debt. As explained in the above articles, however, investors could start demanding higher interest rates if they believe that these deficits increase the risk of holding government bonds.
On the topic of interest rates, Donald B. Marron, a visiting professor at the Georgetown Public Policy Institute, blogs:
According to the IMF, the average maturity of US debt is only 4.4 years. Portugal, Italy, Ireland, and Spain have maturities that are about 50% greater (from 6.2 to 7.4 years), and the UK is almost three times as long at 12.8 years.
The blog concludes:
The short maturity of US debt is a blessing in the short run, since we can benefit from lower interest rates. But it is also poses two risks in the long-run: greater exposure to interest rate increases (if and when they materialize) and a relentless need to ask capital markets to rollover existing debts. Both good reasons why Treasury should continue to gradually extend the maturity of federal borrowing.
The effect of these four factors (bond yield, GDP growth, debt-to-GDP ratio, and primary balance) are also addressed in a Reuter's column titled Sovereign debt maths show risk of vicious circle. The column mentions all four factors in the following excerpt:
To see how these play out, consider two countries. One has a moderate debt load, 50 percent of GDP, which carries a 4 percent average interest rate. If the budget is in primary balance, the government will still run a deficit of 2 percent of GDP, which is 4 percent (the interest rate) of 50 percent (the debt). As long as nominal GDP grows by 4 percent, the ratio of debt to GDP stays the same.
The validity of this final statement (that the ratio to GDP stays the same) can be seen via the following formula:
(new_debt) / (new_GDP) = (i * D - P) / (r * G) = (1.04 * D - 0.0) / (1.04 * G) = D / G
where i = interest rate
D = debt (prior)
P = primary surplus or deficit(-)
r = rate of GDP growth
G = GDP (prior)
The value of 1.04 in the above formula is of course the correct factor to calculate a 4 percent increase and is equal to (4/100) + 1. In any case, the column goes on to describe how this same formula applies to the country with the high debt load of 100 percent of GDP. It then continues:
But the equilibrium is fragile. It can be disturbed in three ways: nominal GDP growth can decline, interest rates can go up or the country can start running a primary deficit. The pain is much worse for highly indebted countries like Greece, which has managed all three at once.
Start with growth. Imagine nominal GDP growth drops to zero. If nothing is done, the debt/GDP ratio will rise by 2 percentage points in the moderately indebted country, but by 4 percentage points in the highly indebted one.
This situation can be represented by the following formula:
(new_debt) / (new_GDP) = (i * D - P) / (r * G) = (1.04 * D - 0.0) / (1.0 * G) = 1.04 * (D / G)
For the moderately indebted country, this will equal 1.04 * 50% of GDP which equals 52% of GDP, an increase of 2% of GDP. For the highly indebted country, this will equal 1.04 * 100% of GDP which equals 104% of GDP, an increase of 4 percent of GDP.
The column continues:
Countries can keep that key ratio from increasing, by running compensating primary surpluses. That means moving from balance to a surplus of 2 percent of GDP for the moderately indebted and from zero to 4 percent for the heavily indebted. The higher the debt level, the more the government’s belt will have to be tightened.
To verify this, one can first calculate the formula for the primary surplus in the case where the debt to GDP ratio does not increase as follows:
(D / G) = (i * D - P) / (r * G) (old debt to GDP ratio equals new debt to GDP ratio)
(D / G) * (r * G) = (i * D - P) (after multiplying both sides by (r * G))
(r * D) + P = (i * D) (after simplifying and adding P to both sides)
P = (i * D) - (r * D) (after subtracting (r * D) from both sides)
P = (i - r) * D (after simplifying)
For the moderately indebted country, the necessary primary surplus will be
P = (1.04 - 1.0) * D = 0.04 * (G/2) = 0.02 * G = 2% of GDP
and for the highly indebted country, the necessary primary surplus will be
P = (1.04 - 1.0) * D = 0.04 * (G) = 0.04 * G = 4% of GDP
Hence, the above formulas show that countries with different levels of debt to GDP can maintain those levels as long as GDP growth keeps pace with the average interest rate and the countries run primary balances of zero. However, they also show that, if one or more of these factors fall out of balance, the country with the higher debt load will see greater negative effects.
The following graph shows the net interest on U.S. Treasury securities as a percentage of outlays, debt, and GDP, along with the nominal growth in GDP:

The actual numbers and sources for this graph can be found at this link. The net interest as a percentage of debt (the blue line) should give the average interest rate being paid on U.S. debt held by the public. As can be seen, the GDP growth rate was generally above the average interest rate from about 1951 to 1981 but was generally below the average interest rate from about 1982 to 2002. From 1951 to 1981, the average interest rate was about 4.2%, nearly half of the average GDP growth rate of about 8.2%. From 1982 to 2002, however, the average interest rate was about 7.3%, over a full percentage point more than the average GDP growth rate of about 6.1%. Hence, it is possible for the average interest rate to surpass the average GDP growth rate for an extended period of time.
The following graph shows the primary and unified surplus or deficit(-) of the U.S. budget:

The actual numbers and sources for this graph can be found at this link. As can be seen, the primary surplus appears to have been, on average, close to balance since 1950, at least until recently. In fact, from 1950 through 2007, the average primary surplus was about 0.14 percent of GDP. However, the graph shows that the primary surplus became a huge deficit in 2009 and is projected to just recover to about a one percent of GDP deficit by 2015. In addition, the tables at this link show that the primary deficit is projected to rise sharply over the coming decades. These numbers come from the most recent U.S. Budget and project that the primary deficit will reach a massive 24.3 percent of GDP by 2085.
Hence, the large primary deficits projected over the long-run would seem the most obvious risk to U.S. sovereign (government) debt. As explained in the above articles, however, investors could start demanding higher interest rates if they believe that these deficits increase the risk of holding government bonds.
On the topic of interest rates, Donald B. Marron, a visiting professor at the Georgetown Public Policy Institute, blogs:
According to the IMF, the average maturity of US debt is only 4.4 years. Portugal, Italy, Ireland, and Spain have maturities that are about 50% greater (from 6.2 to 7.4 years), and the UK is almost three times as long at 12.8 years.
The blog concludes:
The short maturity of US debt is a blessing in the short run, since we can benefit from lower interest rates. But it is also poses two risks in the long-run: greater exposure to interest rate increases (if and when they materialize) and a relentless need to ask capital markets to rollover existing debts. Both good reasons why Treasury should continue to gradually extend the maturity of federal borrowing.
Comments
Post a Comment